알고리즘 문제풀이/백준
[백준/BOJ] 2615번 오목 (C++)
노력의천재
2021. 10. 5. 17:58
https://www.acmicpc.net/problem/2615
2615번: 오목
오목은 바둑판에 검은 바둑알과 흰 바둑알을 교대로 놓아서 겨루는 게임이다. 바둑판에는 19개의 가로줄과 19개의 세로줄이 그려져 있는데 가로줄은 위에서부터 아래로 1번, 2번, ... ,19번의 번호
www.acmicpc.net
그렇게 어렵지 않는 구현 문제였다. 주의할 점이 하나 있는데 오목인지 확인할 때, 다음과 같이 4방향으로 고려해야한다.
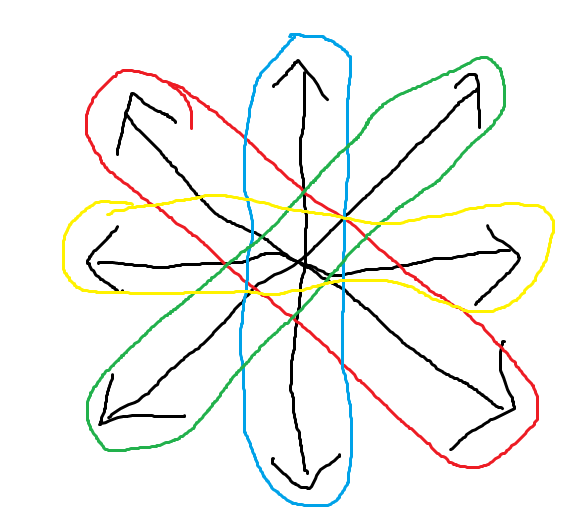
4방향인데, 각각 2번씩 탐색을 하는 것이다. 이렇게 해서 오목이 되는지 확인하면 된다.
#include <iostream>
#include <vector>
#include <queue>
#include <algorithm>
using namespace std;
int map[20][20], win;
int dx[2][4] = {
{-1, -1, -1, 0},
{1, 1, 1, 0}
};
int dy[2][4] = {
{-1, 0, 1, 1},
{1, 0, -1, -1}
};
vector<pair<int, int> > res;
bool flag;
int cmp(pair<int, int> &a, pair<int, int> &b) {
if(a.second == b.second) return a.first < b.first;
return a.second < b.second;
}
void check(int x, int y, int k) {
int pivot = map[x][y];
queue<pair<int, int> > q;
q.push({ x, y });
int nx = x, ny = y;
while(1) {
if(nx + dx[0][k] < 0 || nx + dx[0][k] >= 19 || ny + dy[0][k] < 0 || ny + dy[0][k] >= 19) break;
if(map[nx + dx[0][k]][ny + dy[0][k]] == 0 || map[nx + dx[0][k]][ny + dy[0][k]] != pivot) break;
nx += dx[0][k];
ny += dy[0][k];
q.push({ nx, ny });
}
nx = x, ny = y;
while(1) {
if(nx + dx[1][k] < 0 || nx + dx[1][k] >= 19 || ny + dy[1][k] < 0 || ny + dy[1][k] >= 19) break;
if(map[nx + dx[1][k]][ny + dy[1][k]] == 0 || map[nx + dx[1][k]][ny + dy[1][k]] != pivot) break;
nx += dx[1][k];
ny += dy[1][k];
q.push({ nx, ny });
}
if(q.size() == 5) {
if(pivot == 1) win = 1;
else if(pivot == 2) win = 2;
while(!q.empty()) {
res.push_back(q.front());
q.pop();
}
flag = true;
}
}
int main(void) {
ios_base::sync_with_stdio(false);
cin.tie(0);
for(int i = 0; i < 19; i++) {
for(int j = 0; j < 19; j++) {
cin >> map[i][j];
}
}
for(int i = 0; i < 19; i++) {
for(int j = 0; j < 19; j++) {
if(map[i][j] == 1 || map[i][j] == 2) {
for(int k = 0; k < 4; k++) {
check(i, j, k);
if(flag) {
sort(res.begin(), res.end(), cmp);
cout << win << "\n";
cout << res[0].first + 1 << " " << res[0].second + 1;
return 0;
}
}
}
}
}
cout << win;
}